Karina Kolodina’s work aims to investigate the outcome of the analysis of a heterogeneous neural field model and its homogeneous counterpart, and to get more realistic dynamical models for the brain activities which takes into account microscopic effects.
The PhD thesis is devoted to mathematical neuroscience.
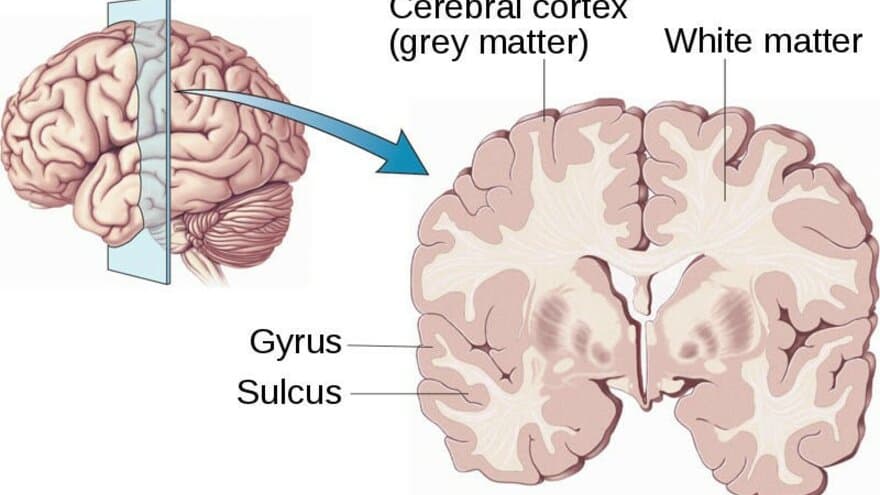
As we know the brain cortex has a microscopic medium. However, in many works it is assumed that the structure is homogeneous and isotropic, which is a simplification of the actual situation. We consider both structures and then compare outcomes. Mathematically neural field models are expressed in terms of integro-differential equations or systems of equations. We therefore analyze the corresponding solutions using different mathematical methods.
Neural field models have had a major impact on the development of our understanding of dynamical activities in the brain cortex. We distinguish between the following dynamical behaviors: spatially and temporally periodic patterns, localized regions of activities (bumps and multi-bumps) and traveling waves (fronts, pulses and spirals) that corresponds to different processes, e.g., visual hallucinations, short term working memory, information processing, orientation tuning in primary visual cortex and control of head direction. Analysis of neural field models also leads to better understanding of different pathological diseases and helps to fight against them.
Neural field models typically take the form of integro-differential equations. Under certain assumptions on firing rates and connectivity functions we study the existence and linear stability of different types of solutions for the models. This is one of our main goals.
One of the traditional neural field models is a continuous model with a homogeneous and isotropic connectivity function. However, it has been shown that the brain cortex has a periodic-like microstucure. This means that we have to incorporate a heterogeneous connectivity to make the model more realistic. One way to take into account the heterogeneity is to apply homogenization theory with the two-scale convergence technique. We then obtain a homogenized neural field model.
The second main aim of this work is to compare results of the analysis of a heterogeneous neural field model and its homogeneous counterpart.